|
THE SCIENCE OF GLOBULAR CLUSTERS
|
As amateur astronomers, we spend a fair bit of our telescope time looking at members of the Messier Objects. Of these, among the easiest – and most popular – classes of deep sky objects to observe with the average amateur telescope are the globular clusters – especially if you’re not at a dark sky site. You all know the sort of thing I mean : who among you hasn’t returned to gaze at M13, M15 or M3 or M5 time and time again. But did you really look at them ? How often did you ask yourselves : What exactly are globular clusters ? How did they form ? Why are they still there ? I’ve been puzzled by globular clusters for a long time. Think about it : what should lots of stars, packed close to each other in a relatively small volume do in a reasonable amount of time - say a few millions years or so ? The laws of gravity require that each star will exert an attraction on all the other members of the cluster, and the cluster should therefore collapse. Remember, globular clusters are old – very old – so they’ve had more than enough time to collapse gravitationally. Yet, they haven’t done so. Why not ? It’s a simple enough question - but what’s the straightforward answer ? Springs !
In order to explain this rather surprising statement we need to first take a look at what globular clusters are, how they formed, and – most importantly – how they behave, both externally and internally. So let’s start with what they are, and what they look like.
In order to explain this rather surprising statement we need to first take a look at what globular clusters are, how they formed, and – most importantly – how they behave, both externally and internally. So let’s start with what they are, and what they look like.
Globular clusters, are each made of hundreds of thousands to a million or so close-packed, gravitationally-bound stars with little or no inter-stellar gas. The clusters measure up to ~100 light years across (depending on the number of stars they contain), and the stars within them are spaced about 1 light year apart : that’s about a quarter of the distance to the nearest star to Earth (Alpha Centauri), and about halfway to the edge of the Oort Cloud that surrounds our Solar System. globular clusters are old, so old that it was once thought they were some kind of primordial feature, as old as the visible universe and as old as the galaxies they are associated with. However, once again, it’s not that simple. They can be anywhere between ~13 to 8 billion years old, which means there has to have been a repeatable, on-going process of globular cluster formation - but what ?
It was once thought that all the stars in a given cluster were of the same type, the same composition and the same age : in other words they all formed at the same time from the same primordial gas cloud. We now know that’s not the case, and this creates a major headache : where did the gas come from to form new, younger stars of different compositions ? Did globular clusters scoop it up from external sources as they orbited galactic centres, or did they produce the gas (and dust ?) internally by the explosion of early-formed stars ? I’m afraid these questions remain unanswered, though scientists have all sorts of opinions on the matter, as we’ll see in a moment.
It was once thought that all the stars in a given cluster were of the same type, the same composition and the same age : in other words they all formed at the same time from the same primordial gas cloud. We now know that’s not the case, and this creates a major headache : where did the gas come from to form new, younger stars of different compositions ? Did globular clusters scoop it up from external sources as they orbited galactic centres, or did they produce the gas (and dust ?) internally by the explosion of early-formed stars ? I’m afraid these questions remain unanswered, though scientists have all sorts of opinions on the matter, as we’ll see in a moment.
In terms of numbers, different galaxies have very different populations of globular clusters. The Milky Way has about 160, but the giant elliptical galaxy M87 has 13,000. Again, no-one knows why. However, here’s another number for you : globular clusters are filled with ultra-dense white dwarf and neutron stars. In fact, the ratio of these dense stars to normal density stars is 100 times greater than in an average galaxy, and it turns out that this is going to be an important factor in explaining why globular clusters don’t collapse on themselves gravitationally – so remember this !
We tend to talk about globular clusters as though they were a homogeneous group, but they’re not. Although most globular clusters can be described in terms of an inner core and an outer halo, it turns out that they show two principle classes of stellar packing patterns, by which I mean how the stars are distributed throughout the volume of the cluster. There are those clusters where the stars are more densely packed in the cluster core – referred to (unsurprisingly) as collapsed core clusters. Then there are those where the stars are more or less homogeneously distributed – known as non-collapsed core clusters. As we’ll see, this is a key observation that helps us understand how globular clusters develop and behave.
We tend to talk about globular clusters as though they were a homogeneous group, but they’re not. Although most globular clusters can be described in terms of an inner core and an outer halo, it turns out that they show two principle classes of stellar packing patterns, by which I mean how the stars are distributed throughout the volume of the cluster. There are those clusters where the stars are more densely packed in the cluster core – referred to (unsurprisingly) as collapsed core clusters. Then there are those where the stars are more or less homogeneously distributed – known as non-collapsed core clusters. As we’ll see, this is a key observation that helps us understand how globular clusters develop and behave.
So, now that we have an idea what globular clusters are, what do they look like ? This image nicely illustrates the range of types of globular cluster. On the left is something so loosely defined that I doubt most of us would recognise it as globular at all, but the professionals do. Left-of-centre is a loosely packed collection of stars that I think we can agree is a globular cluster, but the distinction between core and halo is subtle : it’s there, but it’s subtle. Right-of-centre we see two images of the classical core-and-halo globular cluster structure. Do you see any difference between them ? It’s there, but you have to look for it.
Here’s M15. You can clearly distinguish core and halo here, but while it’s easy to distinguish individual stars in the halo, it’s tough to resolve them in the centre of the bright core. This is what’s referred to as a collapsed-core cluster. There’s an inner part to the core where the stars have begun to gravitationally collapse in toward the centre of the cluster, but they haven’t merged together. Why not ? What stopped them from doing so ?
Now, here’s M54 to re-enforce the point. Once again we can easily distinguish the halo and core, and the collapsed inner core. While we can’t resolve individual stars in the inner core, we can see that the collapse has not gone to completion. Why not ?
Here’s M10, another perennial favourite. Yes, there’s a clear distinction between the dispersed halo and the core. However, notice how individual stars are easily resolvable, even in the centre of the core. This is a typical non-collapsed core cluster. The question is : why hasn’t the core begun to collapse in the manner we saw in the previous two slides ?
We see exactly the same thing in this image of M5, another non-collapsed core cluster with individually resolvable stars even at the centre of the core.
Finally … did you realise that M13 – everyone’s favourite – is a non-collapsed core cluster ? Look at that stellar resolution, right down to the centre of the cluster.
So, now that we know what globular clusters are, and what they look like in detail, we can now ask how globular clusters may have formed. The commonest idea is that they are somehow linked to the early stages of galaxy formation. However, no-one’s sure just how this might have worked. They have also been interpreted as the relict cores of dwarf galaxies captured by larger, growing galaxies. At least, that’s what some say about globular clusters of primitive composition that are aligned in a galactic plane. However, as we’ll see, most globular clusters don’t sit in that plane. Then there’s the idea that they represent super star-clusters that lost their associated gas through a combination of internal stellar winds and external stripping as they passed through the plane of their galaxy. However, the bottom line is that we simply don’t know how they formed : so we’ll just have to take their presence as a given.
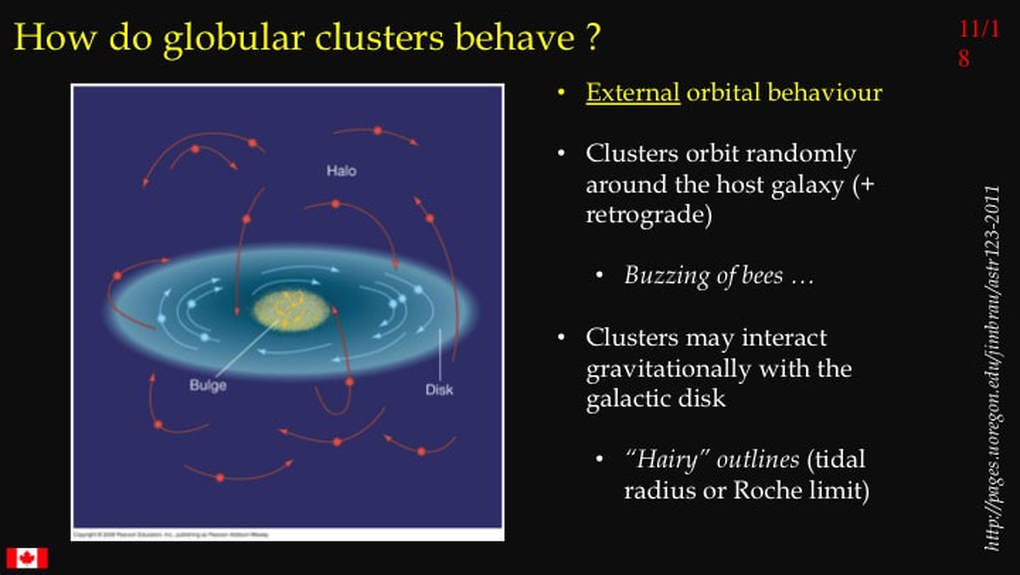
So let’s have a look at how globular clusters behave, beginning with their external orbital behaviour. First, we need to know that globular clusters occur in three places in galaxies : (i) as an external halo, (ii) within the galactic disk and (iii) within the galactic bulge. Unlike the stars of a galactic disk, globular clusters located in the halo and the bulge do not orbit systematically around the galactic centre. Their orbits and orbital directions are randomised : they go every which way. Occasionally, globular clusters from the halo will pass through the galactic plane where they interact gravitationally with the stars of the galactic disk. This leads to stars in the outer parts of the cluster halo (beyond the cluster’s Roche limit) being tidally torn away from the cluster. This explains the fuzzy, “hairy” appearance of many globular clusters that commonly show external strands of stars only loosely bound to the main cluster.
Now let’s turn our attention to how globular clusters behave internally. This is where things get interesting and a tad complicated, but we’ll get through this together. It’s a simple fact of Physics that self-gravitating systems with random internal motions - like globular clusters - cannot attain stable gravitational equilibrium. In the inner cores of globular clusters, the spacing of stars is of the order of the distance from the Sun to Pluto. That’s pretty close-spaced ! If the stars are simple and randomly moving in space, gravity dictates that the cluster must collapse on itself - and pretty fast too ! So what is preventing this inevitability ? Gravity itself. Bear with me and I will explain.
All stars in a globular cluster interact gravitationally with their nearest neighbours, but also with all the other stars of the cluster. Because of this, cosmologists can analyse the internal behaviour of stars in globular clusters by treating them as they treat atoms in a gas : statistically - something we understand very well. Atoms in a gas are always moving – except at absolute zero – and move randomly, i.e. in every direction possible. In a globular cluster, stars also move randomly. Even if they started out circular around a cluster centre, stellar orbits become randomised with time. I’ll explain how in just a moment, but what this means is that the circular component of stellar motion around the cluster progressively diminishes as the radial component of stellar motion in and out of the cluster increases. In other words, the stars internal to globular clusters are buzzing randomly like a swarm of bees. There’s a consequence to this randomisation of orbits : globular clusters do not rotate. There are a few exceptions (e.g. M13), but their bulk rotation is so slow that it only serves to indicate that individual stellar orbits within them were not always as randomised as we see them now.
In addition to their randomised internal motions, stars within a globular cluster undergo what’s known as “mass segregation” whereby the larger and/or denser stars (i.e. the heavier ones – remember all those white dwarf and neutron stars ?) migrate toward the inner part of the cluster - and the lighter stars migrate outwards. We’ll see why in just a moment.
OK, so globular clusters do not rotate, they are internally stratified according to stellar mass, and their constituent stars buzz around at random. How does all this happen, and what does it have to do with stopping globular clusters from collapsing gravitationally ?
All stars in a globular cluster interact gravitationally with their nearest neighbours, but also with all the other stars of the cluster. Because of this, cosmologists can analyse the internal behaviour of stars in globular clusters by treating them as they treat atoms in a gas : statistically - something we understand very well. Atoms in a gas are always moving – except at absolute zero – and move randomly, i.e. in every direction possible. In a globular cluster, stars also move randomly. Even if they started out circular around a cluster centre, stellar orbits become randomised with time. I’ll explain how in just a moment, but what this means is that the circular component of stellar motion around the cluster progressively diminishes as the radial component of stellar motion in and out of the cluster increases. In other words, the stars internal to globular clusters are buzzing randomly like a swarm of bees. There’s a consequence to this randomisation of orbits : globular clusters do not rotate. There are a few exceptions (e.g. M13), but their bulk rotation is so slow that it only serves to indicate that individual stellar orbits within them were not always as randomised as we see them now.
In addition to their randomised internal motions, stars within a globular cluster undergo what’s known as “mass segregation” whereby the larger and/or denser stars (i.e. the heavier ones – remember all those white dwarf and neutron stars ?) migrate toward the inner part of the cluster - and the lighter stars migrate outwards. We’ll see why in just a moment.
OK, so globular clusters do not rotate, they are internally stratified according to stellar mass, and their constituent stars buzz around at random. How does all this happen, and what does it have to do with stopping globular clusters from collapsing gravitationally ?
What we’ve looked at so far is how the stars in a globular cluster interact gravitationally en-masse. Let’s now look at how they interact gravitationally as individuals. Remember, cosmologists treat the stars in globular clusters by analogy with the atoms in a gas. This has a major consequence. The motion of atoms in a gas is a function of temperature : in other words a function of heat. At absolute zero, there is no heat and atoms in a gas do not move (they don’t even vibrate). However, motion in a globular cluster is also a function of gravity, so cosmologists describe core collapse as a gravothermal or a gravokinetic instability - or eventually catastrophe. Remember, “kinetic” just means movement or motion.
What’s another very well known example of a gravothermal or a gravokinetic system – one that you all know very well, but this time one that’s in equilibrium ? The Sun. The gravity due to the Sun’s mass is trying to collapse the Sun towards it centre, while the heat of the Sun – in other words the kinetic motion (pressure) of its constituent hydrogen and helium – is trying to push the mass outwards.The equilibrium between these two sets of forces gives us the Sun as we see it now. So cosmologists encourage us to see the internal behaviour of globular clusters through an analogy with the Sun. So let’s do just that. The gravitational component of our globular cluster model is easy : stars want to gravitationally pull each other into the centre of the cluster. Our simple, straight forward question is : why doesn’t this happen – why don’t globular clusters collapse ? From our analogy with the Sun, we now need to look at the thermal component of a globular cluster, the component that’s working against gravitational collapse of the cluster as a whole.
What do we mean by “thermal” in the context of a globular cluster ? We mean the motion of the stars – the internal kinetic energy of the cluster. Using our gas analogy, it turns out that there’s a simple relationship between kinetic energy on the one hand and the mass and velocity of stars on the other - when stars interact individually ... and yes, it can be applied to gravitationally interacting pairs of stars. In gases, particles bounce off each other. In clusters, stars swing by each other and exchange energy by gravitational interaction. The simple relationship states that the bulk kinetic energy (Ke) of the gravitationally interacting pair of stars will be equally divided between the two stars when they separate and go their own way such that each star’s mass (M) multiplied its velocity (v) is the same. If two stars of different mass interact gravitationally - because M times v for each star must be equal to that for the other star - more massive stars must slow down, and less massive stars must speed up. This is why globular clusters segregate according to stellar mass : slow, massive stars migrate in towards the cluster core, while faster, less massive stars migrate out into the cluster halo. Pretty neat, but now we have a problem. If slower, more massive stars are concentrating in the cluster core, then gravity should now accelerate core collapse. We should get runaway collapse and a real catastrophe !
What’s another very well known example of a gravothermal or a gravokinetic system – one that you all know very well, but this time one that’s in equilibrium ? The Sun. The gravity due to the Sun’s mass is trying to collapse the Sun towards it centre, while the heat of the Sun – in other words the kinetic motion (pressure) of its constituent hydrogen and helium – is trying to push the mass outwards.The equilibrium between these two sets of forces gives us the Sun as we see it now. So cosmologists encourage us to see the internal behaviour of globular clusters through an analogy with the Sun. So let’s do just that. The gravitational component of our globular cluster model is easy : stars want to gravitationally pull each other into the centre of the cluster. Our simple, straight forward question is : why doesn’t this happen – why don’t globular clusters collapse ? From our analogy with the Sun, we now need to look at the thermal component of a globular cluster, the component that’s working against gravitational collapse of the cluster as a whole.
What do we mean by “thermal” in the context of a globular cluster ? We mean the motion of the stars – the internal kinetic energy of the cluster. Using our gas analogy, it turns out that there’s a simple relationship between kinetic energy on the one hand and the mass and velocity of stars on the other - when stars interact individually ... and yes, it can be applied to gravitationally interacting pairs of stars. In gases, particles bounce off each other. In clusters, stars swing by each other and exchange energy by gravitational interaction. The simple relationship states that the bulk kinetic energy (Ke) of the gravitationally interacting pair of stars will be equally divided between the two stars when they separate and go their own way such that each star’s mass (M) multiplied its velocity (v) is the same. If two stars of different mass interact gravitationally - because M times v for each star must be equal to that for the other star - more massive stars must slow down, and less massive stars must speed up. This is why globular clusters segregate according to stellar mass : slow, massive stars migrate in towards the cluster core, while faster, less massive stars migrate out into the cluster halo. Pretty neat, but now we have a problem. If slower, more massive stars are concentrating in the cluster core, then gravity should now accelerate core collapse. We should get runaway collapse and a real catastrophe !
So why doesn’t this happen ? What prevents it ? Binary star systems that act as springs ! Binary stars are very common in globular clusters. According to studies of X-ray binaries, they are 1000 times more common than in an average galaxy, and may make up 10-20% of the stars in a globular cluster. Why X-ray binaries ? Because of all those white dwarf and neutron stars I told you about earlier. Because binary systems are more massive than average individual stars, they participate in the mass segregation process that makes “heavy” stars migrate preferentially into the cluster core, where they play a key role in preventing core collapse.
How do binary stars act like springs ? Let’s deal with that in a moment. For now, let’s just say that they dynamically heat – or increase the kinetic energy of - the core of the globular cluster. Here’s how … When a third star gravitationally interacts with a binary star system in the core of a globular cluster, there’s an exchange of energy - gravitational energy that can convert to kinetic energy. The internal orbits of the binary stars around their common centre of gravity contract as the binary passes kinetic energy to the passing star, which then speeds up and heads out on a random trajectory towards the cluster halo – leading to orbit randomisation. There’s also a slowing of the binary’s bulk motion, which enhances its tendency to migrate even deeper into the cluster core. It also turns out that the tighter the orbit of binary stars about their common gravitational centre, the more energy they are able to transfer to the next passing star. In short - they get energetically “wound up” - which is where the spring analogy comes from.
At last, we now have a mechanism that can enhance the kinetic energy of individual stars, speeding them up and kicking them out of the cluster core, effectively slowing – perhaps stopping – core collapse. This is also the mechanism that randomises individual stellar orbits within the cluster as a whole. In fact, some cosmologists think that as the density of the cluster core approaches a critical value, there will be so many binaries that the core collapse does more than just slow down, it bounces and the core expands again to start another cycle of core collapse as the binaries get further apart !
How do binary stars act like springs ? Let’s deal with that in a moment. For now, let’s just say that they dynamically heat – or increase the kinetic energy of - the core of the globular cluster. Here’s how … When a third star gravitationally interacts with a binary star system in the core of a globular cluster, there’s an exchange of energy - gravitational energy that can convert to kinetic energy. The internal orbits of the binary stars around their common centre of gravity contract as the binary passes kinetic energy to the passing star, which then speeds up and heads out on a random trajectory towards the cluster halo – leading to orbit randomisation. There’s also a slowing of the binary’s bulk motion, which enhances its tendency to migrate even deeper into the cluster core. It also turns out that the tighter the orbit of binary stars about their common gravitational centre, the more energy they are able to transfer to the next passing star. In short - they get energetically “wound up” - which is where the spring analogy comes from.
At last, we now have a mechanism that can enhance the kinetic energy of individual stars, speeding them up and kicking them out of the cluster core, effectively slowing – perhaps stopping – core collapse. This is also the mechanism that randomises individual stellar orbits within the cluster as a whole. In fact, some cosmologists think that as the density of the cluster core approaches a critical value, there will be so many binaries that the core collapse does more than just slow down, it bounces and the core expands again to start another cycle of core collapse as the binaries get further apart !
So let’s summarise what we’ve just looked at, keeping in mind the solar analogy wherein gravity tries to collapse the Sun, but the internal heat and kinetic energy of the Sun successfully prevents this from happening - at least for now ! Binary star systems transfer kinetic energy to passing third stars by gravitational energy transfer. That transferred kinetic energy can be seen as “heat”. In fact, this process is technically referred to as “binary burning”. In the cluster core, that “heat: leads to cluster core expansion as stars speed up and migrate out to the halo. However, that core expansion leads to a decrease in the density of the core (stars further apart), and a decrease in the total kinetic energy of the cluster core, which in turn leads to renewed collapse. Hence, some cosmologists wonder if globular clusters are forever oscillating between collapsed and non-collapsed core states, which would mean that when we observe a globular cluster, random chance dictates what physical state we observe it in.
Well, this sounds great ! We’ve got a model that seems to explain it all : random stellar orbits and mass segregation in globular clusters - and why globular clusters don’t gravitationally collapse on themselves. It even explains why some clusters show evidence for core collapse, while others don’t. However, we still have a major problem. As individual binary star systems exchange kinetic energy with passing stars, their binary orbits around their common gravitational centre shrink. So, after ~13 billion years, the original binaries in a globular cluster should have disappeared as their constituent stars merged. Hence, even if they had been able to slow globular cluster collapse, it should’ve started again a long time ago. So why are the globular clusters still there ?
This was a major headache for cosmologists until they found a viable solution : secondary binary star systems ! The general idea is that in the densely packed core of globular clusters, random interactions between single stars will lead to the formation of new secondary binary star systems to replace the primary ones that will have evolved – perhaps into neutron stars. Hence the kinetic “heat” engine is maintained within the globular cluster … and core collapse is forever delayed or stopped.
This was a major headache for cosmologists until they found a viable solution : secondary binary star systems ! The general idea is that in the densely packed core of globular clusters, random interactions between single stars will lead to the formation of new secondary binary star systems to replace the primary ones that will have evolved – perhaps into neutron stars. Hence the kinetic “heat” engine is maintained within the globular cluster … and core collapse is forever delayed or stopped.
In closing let’s ask the question cosmologists are asking themselves right now : “if they don’t collapse, what is the ultimate fate of globular clusters ?” It turns out that if you push the model we’ve just looked at far enough in time, the speeding up of stars and their transfer from the cluster core to the cluster halo will eventually lead to stars leaving the halo altogether. If it happens quickly it’s referred to as ejection. If it happens slowly it’s called evaporation of the cluster. Either way, the time frame is longer than that of the visible universe : so don’t expect to observe it in action any time soon !
If you want to see a more extensive and more detailed presentation I gave in the Ottawa Astronomy Workshop Series : see https://www.youtube.com/watch?v=EpkW68NUFE
If you want to see a more extensive and more detailed presentation I gave in the Ottawa Astronomy Workshop Series : see https://www.youtube.com/watch?v=EpkW68NUFE
Proudly powered by Weebly